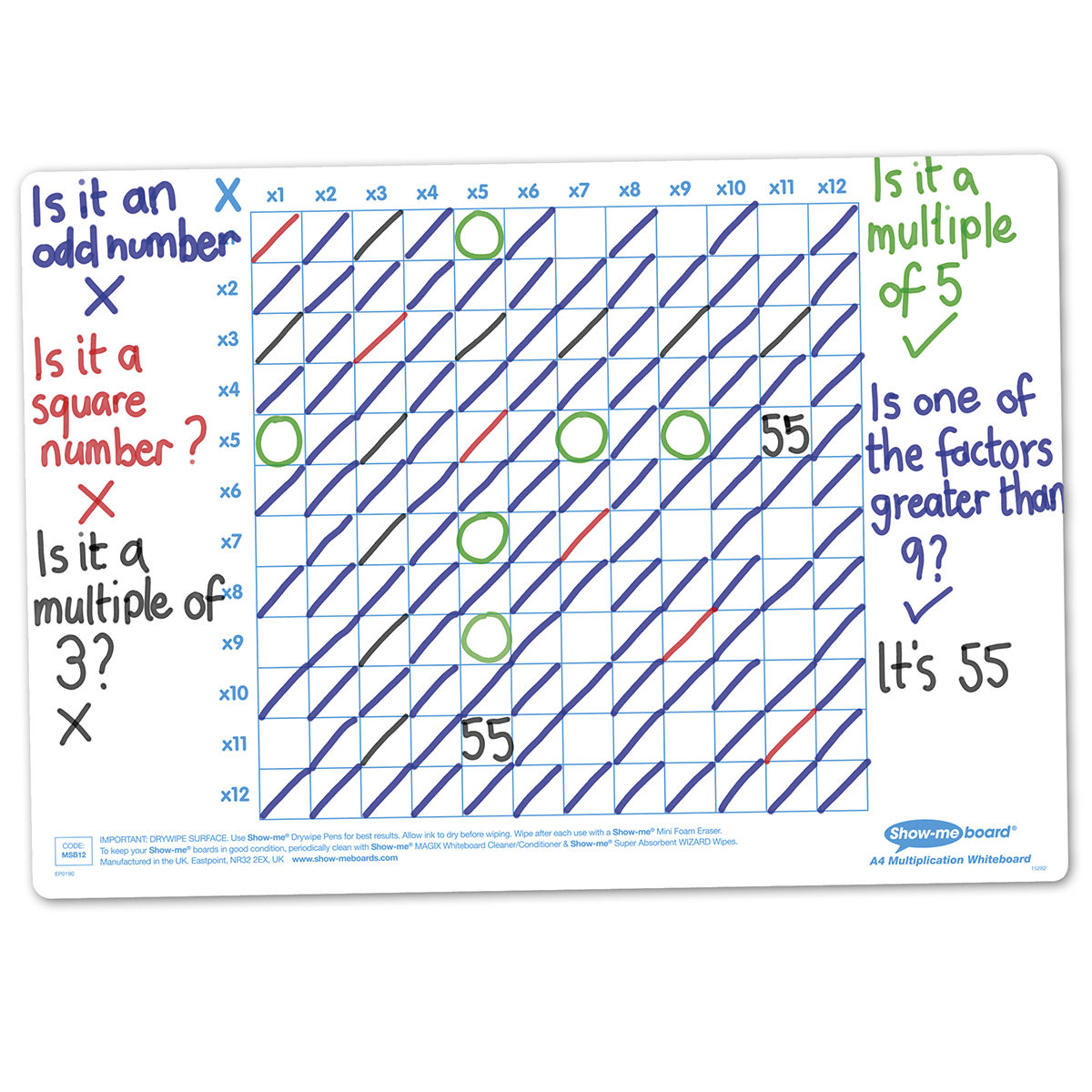
Player 1 secretly thinks of a number that would appear within the multiplication grid.
Player 2 then asks Player 1 questions about their chosen number that they can only answer with ‘yes’ or ‘no’.
Player 2 uses the blank multiplication grid to keep track of the answers.
For example, If Player 2 asked ‘Is your number even?’ and Player 1 responded ‘yes’, Player 2 might cross off all the odd numbers that would appear on the multiplication square.
Player 2 can only ‘guess the number’ when they are left with 2 possibilities.
Once Player 2 has correctly guessed the number, swap roles.
Who can find the number using the least number of questions?